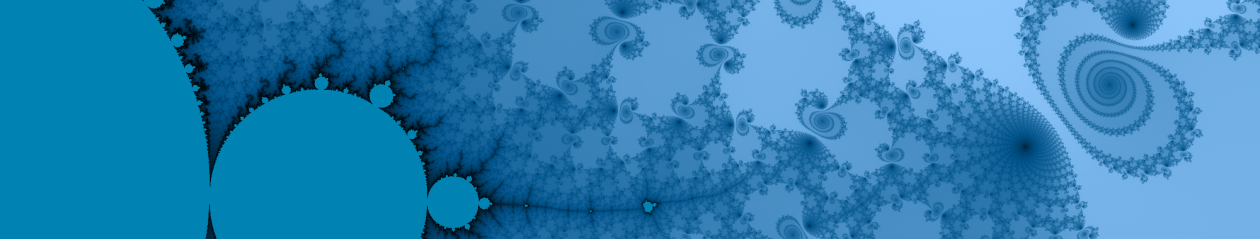
In case anyone has not already noticed, I love fractals.
I’ve been a few years (two decades!) perfecting my program to paint the Mandelbrot set, but I’m never totally satisfied. There are still many details to polish and I’m always lacking the same ingredient: time!
What I can share is a few samples of the images it can generate. There goes the first one:
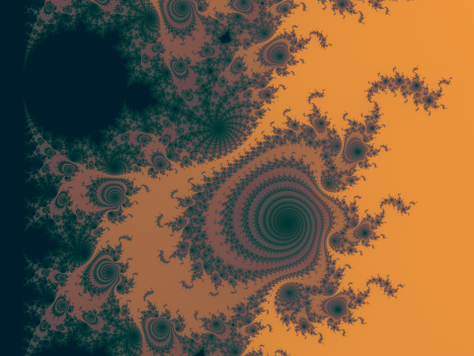
Download in giant format (beware: 12’6 MB)
Wondering what the Mandelbrot set is?
Thousands of web pages and books try to explain it, but I’ll take the opportunity to mention my book, which contains, among other adventures, my best attempt at explaining it.
For the mathematicians:
The Mandelbrot set is the set of points of the complex plane which, used as constant C in the series Zn+1=Zn2+C , where Z0=0 , do not make the length (the Euclidean norm) of Z tend to infinity. The previous image represents a small region of the complex plane. Almost all the points painted in dark blue (at the left) belong to the Mandelbrot set. The points painted in other colours do not belong to the Mandelbrot set. For these latter points, the colour was chosen depending on the n from which the length of Zn is greater than 2. When this happens, we know that the series will tend to infinity, so we stop calculating it. When this doesn’t happen, since we can’t compute indefinitely, we must fix a maximum value for n. From that moment, if the length of Zn didn’t exceed the value 2, we paint the pixel in dark blue and proceed to compute another pixel.
In fact, if I had read the previous paragraph when I had not yet heard of fractals, I would have stayed exactly like before reading it.
For the rest of mortals:
The Mandelbrot set is a mathematical object, just like the circle, the square and the dodecahedron. Nothing more, nothing less. It belongs to Plato’s world of Ideas (or world of forms). As the rest of mathematical things, it doesn’t need a material universe to “be”. It simply “is”. It’s the material world which seems to work according to mathematical laws.
Perhaps this last statement sounds ridiculous to you. Our culture is full of memes ridiculing the scientist who aspires to calculate everything, to explain everything, to predict the future. I’m not really sure if scientists had such pretensions a century ago, or if there is rather an almost vindictive interest to discredit science. The exaggerated faith in science might seem ridiculous from outside. Seen from the outside, any exaggerated faith seems ridiculous. Especially from the point of view of another faith.
The point is that the mistake was thinking that, if the universe obeys mathematical laws, we’ll be able to explain everything and predict the future. Nothing could be further from reality. In fact, nothing could be further from mathematics. There are a few mathematical truths with a huge transcendental importance, which were discovered in the last century, but have only penetrated our culture as mere anecdotes.
One of these transcendental truths is chaos theory. Perhaps you know about it as the “butterfly effect” but that’s just the anecdote, the shocking example used to try to explain something bigger: that to predict the future we’d have to make an overwhelming amount of calculations, and before that we’d need to know the present at an overwhelming level of detail. So overwhelming, that the most efficient way to predict the future accurately is to sit and wait for it. That is, any machine that calculated the future would be bigger and require more time to try to predict it that the material world itself.
Actually it is not a new idea with respect to the material world. It is what humanity has experienced since it walks the Earth. It is the wall blocking the path of those who seek to calculate the future accurately. The novelty is that this also happens in Plato’s world of Ideas. Unpredictability is not due to anything of the material world nor any other metaphysical and unfathomable world we wish to invent. Unpredictability is inherent in the ideal world of mathematics.
Enough for now. There goes another image:
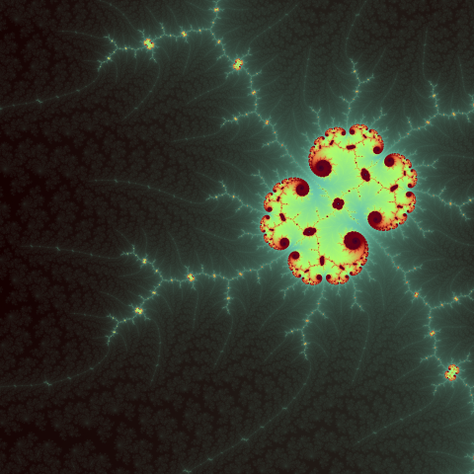
Download in giant format (beware: 19’2 MB)
In this second picture there hardly is any area that really belongs to the set. They are only a handful of white pixels here and there. In the reduced version you can’t even see them. However, the Mandelbrot set is connected. That is, these small “islands” in the set area are connected by very fine filaments which also belong to the set. These filaments are so twisted that the boundary of the Mandelbrot set has an infinite length. It is a curious fact, since the area of the set is finite.
In this picture we almost see the filaments as pale green lightnings. What we see are not really the filaments themselves, which in many points are infinitely thin. What we see shine are the points close to them. With these values of C, the series takes longer to step out of the circle of radius 2.
Returning to the subject of unpredictability, I’ll present an example:
We know of many points that belong to the Mandelbrot set. We know it with absolute certainty because, with these values of C, the sequence is cyclic. The easiest example is C=0. With this value of C, every value of Z is zero. Another example is C=-1, with which the series oscillates indefinitely between -1 and 0. And yet another example is C=-2, with which the series soon gets stuck at value Z=2.
We also know that, for every C whose length is greater than 2, the series tends to infinity. For C=3, for instance, the values of Z are: 0, 3, 12, 147, 21612, 467078547, 218162369067631000… I will not go into the details, but it can be proven and it is reasonably intuitive.
Nevertheless, for many of the infinite points inside the circle of radius 2, we don’t have a general method to predict in every case whether the series will tend to infinity or not. The only way to find it out is calculating the series, and this is not always in our hand. In many cases, as the calculation progresses, the numbers have more and more digits.
If any value of Z steps out of the circle of radius 2, it’s done: this C does not belong to the set. On the other hand, if any value of Z is exactly equal to a previous one, it’s done: the series cycles, and this value of C does belong to the set. But, what happens if we go on calculating and none of this things happen? We’ll have to stop at some point. We can’t compute indefinitely!
The theory of chaos states that there are mathematical systems that exhibit this behaviour. They are governed by perfectly defined mathematical laws, but the slightest variation in the initial conditions can decisively affect the outcome much later. Furthermore, there’s no shortcut to predict those behaviour changes. The only way is to calculate everything step by step with absolute precision. So much precision that it escapes our possibilities because it gets infinitely larger than the system we wanted to predict in first place. Translated to a material universe ruled by these laws: the only way to predict the future with absolute precision is to sit and wait for it.
Concluding…
All of this sets a limit to what we can calculate and predict, but it poses no obstacle for the universe being a lot of matter/energy set to spin, obeying with absolute precision some predefined mathematical and physical laws, without any outside influence after the Big Bang.
The question is: can some mathematical and physical laws breed everything we see around us?
In this sense, the Mandelbrot set and other fractals are a surprising discovery. They are purely mathematical objects, like the circle or the dodecahedron, yet their shapes resemble living tissues and landscapes.
All of this and more is wonderfully explained in a beautiful BBC documentary called “The Secret Life of Chaos”. It can be easily found on Youtube.
There goes the last image. Of the three, this is the one I like most:
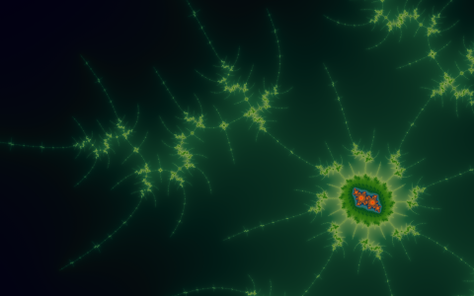
Download in giant format (beware: 23’3 MB)
One last clarification: these images are in part a creation of mine, but that part is very small. The only creative act is the choice of the colours. The shapes are already there. They simply “are”, like the roundness of a circle “is” without the need of me drawing it or even imagining it.
There’s other part in the generation of these images that might look like a creative act, but it’s only discovery: the choice of which part of the Mandelbrot set to zoom into and compute. The Mandelbrot set is there with its infinite complexity, with its infinite filaments and whirlpools. It simply “is”, without the need of me computing it.